
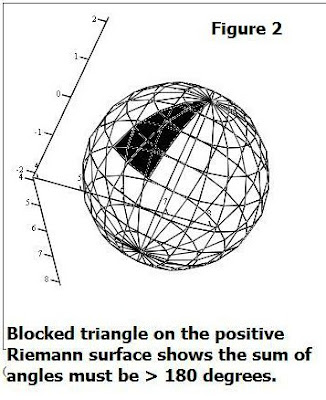
When a surface has a constant zero Gaussian curvature, then it is a developable surface and the geometry of the surface is Euclidean geometry. Most surfaces will contain regions of positive Gaussian curvature (elliptical points) and regions of negative Gaussian curvature separated by a curve of points with zero Gaussian curvature called a parabolic line. If one of the principal curvatures is zero: κ 1 κ 2 = 0, the Gaussian curvature is zero and the surface is said to have a parabolic point.Because one principal curvature is negative, one is positive, and the normal curvature varies continuously if you rotate a plane orthogonal to the surface around the normal to the surface in two directions, the normal curvatures will be zero giving the asymptotic curves for that point. At such points, the surface will be saddle shaped. If the principal curvatures have different signs: κ 1 κ 2 All sectional curvatures will have the same sign. At such points, the surface will be dome like, locally lying on one side of its tangent plane. If both principal curvatures are of the same sign: κ 1 κ 2 > 0, then the Gaussian curvature is positive and the surface is said to have an elliptic point.The sign of the Gaussian curvature can be used to characterise the surface. The Gaussian curvature is the product of the two principal curvatures Κ = κ 1 κ 2. For most points on most “smooth” surfaces, different normal sections will have different curvatures the maximum and minimum values of these are called the principal curvatures, call these κ 1, κ 2. The intersection of a normal plane and the surface will form a curve called a normal section and the curvature of this curve is the normal curvature. Saddle surface with normal planes in directions of principal curvaturesĪt any point on a surface, we can find a normal vector that is at right angles to the surface planes containing the normal vector are called normal planes.


 0 kommentar(er)
0 kommentar(er)
